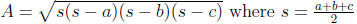Algebra Study Guide
4.1 Polynomial Functions and their Graphs
• End behavior:
◦ Look at leading coefficient/exponent and check sign
◦ If polynomial is factored, check sign of each factor and multiply
• Graphing a polynomial:
◦ Factor
◦ Find x- and y-intercepts
◦ Find end behavior
◦ Either use test points between the intercepts or memorize the shape around
zeros
depending on the multiplicity:
— If multiplicity is 1, then it crosses the x-axis in a
straight line
— If multiplicity is even, then it turns back around
— If multiplicity is odd > 1, then it ”squiggles” through the x-axis
4.2 Dividing Polynomials
• Long Division: Make sure to fill in missing powers
• Synthetic Division: Only works for division by (x − c).
Again make sure to fill in 0’s
for missing powers
• Remainder Theorem: to find P(c) carry out a synthetic
division for c, the remainder
is P(c)
• Factor Theorem: c is a zero of P ↔ (x − c) is a factor of P(x)
4.3 Real Zeros of Polynomials
• Rational Zeros Theorem: The possible rational zeros of a polynomial are of
the form
p/q where p is a factor of the constant coefficient a0 and q is a factor of the
leading
coefficient an
• How to find all zeros of a polynomial:
◦ Try previous factoring methods like substitution or
grouping, if this does not work
then:
◦ List all possible rational zeros using the Rational Zeros Theorem
◦ Test the possible zeros
◦ If you find a zero, factor it out
◦ Repeat from the top until your polynomial is quadratic, then factor/complete
the
square/quadratic formula
4.4 Complex Zeros and the Fundamental Theorem of
Algebra
• Fundamental Theorem of Algebra: every polynomial of degree n has precisely
n zeros
(zeros of multiplicity k are counted k times)
• Conjugate Zeros Theorem: If a complex number is a zero
of polynomial with real
coefficient, then its conjugate is also a zero
4.5 Rational Functions
• Horizontal asymptotes: n is the degree of the numerator, m is the degree
of the denominator
◦ n > m: no horizontal asymptote
◦ n = m: horizontal asymptote is
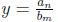
◦
n < m: horizontal asymptote is y = 0
• Vertical asymptotes: zeros of the denominator (that do not cancel with the numerator)
• Graphing rational functions:
◦ Factor numerator and denominator
◦
Find x- and y-intercepts
◦
Find horizontal and vertical asymptotes
◦
Either use test points between intercepts/vertical asymptotes or use the shape
around vertical asymptotes/intercepts to determine the shape of the graph
• Slant asymptote: only exists if the degree of the
numerator is one greater than the
degree of the denominator: use long/synthetic division
5.1 Exponential Functions
• f(x) = ax, memorize the graph:
◦ Horizontal asymptote y = 0
◦
no vertical asymptote
◦
Domain = (−∞,∞)
◦
Range = (0,∞)
• Compound interest formula:
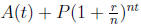
• Continuously compounded interest:
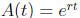
5.2 Logarithmic Functions
• Definition of logarithm: logb a = x ↔ bx = a
• Properties:
◦ logb 1 = 0
◦
logb b = 0
◦
logb bx = x
◦
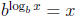
• f(x) = logb x, memorize the graph:
◦ Vertical asymptote: x = 0
◦
no horizontal asymptote
◦
Domain = (0,∞)
◦
Range = (−∞,∞)
• Finding the domain of logarithmic function: logarithms only defined for positive numbers
• Common log:
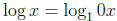
• Natural log:
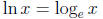
5.3 Laws of Logarithms
• logb(xy) = logb x + logb y
•
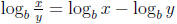
•
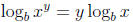
• no laws for logb(x + y) or logb x · logb y
• Change of base:
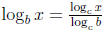 where c can be any positive number
where c can be any positive number
5.4 Exponential and Logarithmic Equations
• Solving exponential equations:
◦
Isolate the exponential term on one side
◦
Take logarithm of both sides:
— If there is only one exponential term, use that base for the log
— If there is an exponential term on both sides, use either the common or
natural
log
◦ Pull the exponent to the front and solve the equation
• Solving logarithmic equations:
◦
If there are multiple logarithmic terms, combine them into one using logarithmic
laws
◦
Isolate the logarithmic term on one side
◦
Raise the base of the logarithm to the left and the right side of the equation
◦
Use the property
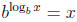 to get rid of the log
to get rid of the log
◦
Solve the equation
• Two special cases of exponential equations:
◦
Combination of exponential and polynomial terms: try to factor
◦
Sum of multiple exponential terms: try to use substitution
6.1 Angle Measure
• Relationship between Degrees and Radians:
◦
convert from degrees to radians by multiplying by
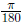
◦ convert from radians to degrees by multiplying by
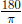
• Coterminal angles: Angle between 0° and 360° degrees (or 0 and 2π )
• Length of a circular arc: s = rθ (θ in rad)
• Area of a circular sector:
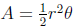 (θ in rad)
(θ in rad)
• Linear Speed and Angular Speed:
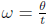 and
and
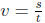
• Relationship between linear and angular speed: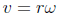
6.2 Trigonometry of Right Triangles
• Trigonometric Ratios:
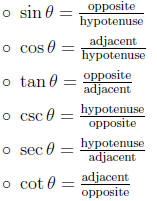
• Values of the trig ratios for angles 30° , 45° and 60°
• Solving right triangles
6.3 Trigonometric Functions of Angles
• Memorize in which quadrants each trig function is positive
• Reference angles: Acute angle formed by x-axis and terminal side
• Using reference angles to evaluate trig functions
• Reciprocal Identities:
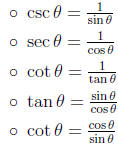
• Pythagorean Identities:
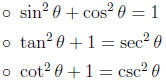
• Expressing trig functions in terms of other trig functions
• Evaluating trig functions using identities
• Area of a Triangle: 1/2ab sinθ (where θ is the angle between a and b)
6.4 Law of Sines
• Law of Sines: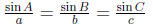
• Solving triangles:
◦
SAA
◦
SSA (either no solution, one solution or two solutions)
6.5 Law of Cosines
• Law of Cosines:
◦
a2 = b2 + c2 − 2bc cosA
◦
b2 = a2 + c2 − 2ac cosB
◦
c2 = a2 + b2 − 2ab cosC
Solving triangles:
◦
SSS
◦
SAS
• Navigation: Bearing
• Heron’s Formula: Area of a triangle is